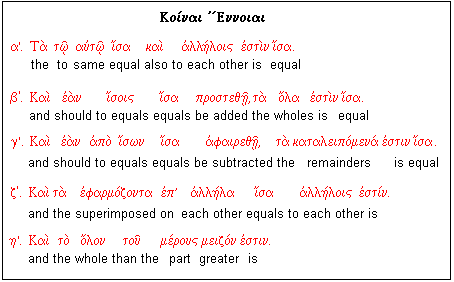
The axioms were called "common notions" or koi/nai e/)nnoiai by Euclid. Aristotle dignified them as a)xiw/mata or "worthy things". These are simply agreements about how the common notions of equality, greater than, and less than act in practice, and are not part of geometry, but more or less common to all mathematics. There has been little contention over them, but commentators have felt it desirable to add extra axioms to the five that satisfied Euclid. These additional axioms cover adding or subtracting equals or unequals, doubles or halves, to quantities, and are quite superfluous. There was also a ninth axiom that two straight lines could not enclose a space. The five Euclidean axioms are given in the box below, as usual with literal translations.

The axioms are easy enough to read that at least some often appear in an introductory Greek course. They show clearly how Greek uses a singular verb for a plural neuter subject; in the box, I have steadfastly used "is" to make this stand out. As usual, e)a/n calls for the subjunctive, and this is seen in the verbs ending with hi. After the long vowel, the ι goes subscript. Both forms are aorist passive subjunctive. The verbs are from prosti/qhmi, "add to", and a)faire/w, "take away". The accent on mei/zon is unusual; it is attracted to the ultima by the following enclitic.
With the definitions, postulates and axioms established, we are now ready to tackle the demonstrations of the propositions. Each step must be justified by reference to these principles, and to propositions already proved by their aid. We have already seen the first proposition, which is the problem of constructing an equilateral triangle on a given line, in an earlier lesson. It would be good to review this proposition, and to justify each step. We do not have room to go through the whole of the Elements, but we shall show some of the most important propositions, and give some idea of Greek mathematics beyond the Elements.
Composed by J. B. Calvert
Created 17 September 2000
Last revised