Optoelectronics
Review of Semiconductors
Semiconductors can create and detect electromagnetic radiation. This radiation carries energy, so it can join conduction currents as part of our electronic circuits. The eye is sensitive to electromagnetic radiation with wavelengths between 400nm and 700nm, and such radiation is called light. I'll simply call all radiation light in what follows, although it may include radiation to which the eye is not sensitive. Light has only 5 letters, while electromagnetic radiation has 26, including the space.
The frequency of light is connected to its wavelength by λf = c, where c is the phase velocity of light, 2.9979 x 108 m/s (now an arbitrary constant defining the metre), f the frequency in Hz, and λ the wavelength in metres. The frequency is extremely high, so it is more convenient to use wavelegth, usually in nanometres. As we have said, visible light is in the range 400nm (blue) to 700nm (red). The sensitivity of the eye peaks at 550nm (green), falling off smoothly at both limits. We will be concerned with the visible, and with radation of wavelength greater than 700nm, called infrared. At the other end is the ultraviolet, with shorter wavelengths. The deep blue at the short-wavelength end of the visible spectrum was called violet for some reason; indigo would be better--violet it is not. Then we would have had the ultraindigo.
When light interacts with atomic systems, energy is exchanged only in discrete amounts depending on the frequency of the light. The fundamental amount, or quantum, is E = hf, where h is Planck's constant, 6.6261 x 10-34 Joule-seconds. A friendlier energy unit is the electron-volt, eV, the energy given to an electron by a potential difference of one volt, equal to 1.602 x 10-19 J. In terms of wavelength, the quantum energy is E = 1240/λ eV. For the visual range, this is from 3.1 eV to 1.8 eV. The quantum energy in the ultraviolet is large enough to start smashing molecules, so ultraviolet is bad for you. There is no such problem in the infrared.
A semiconductor is characterized by an energy gap between the electrons that have crowded into all the available places (a band)and so act as if they cannot move because there is no place to go, and the wide-open spaces where the electrons can freely go where they will. N-type semiconductors have extra electrons added to the wide-open spaces by impurities that can easily supply them, and p-type semiconductors have holes that can move around created by impurities that can capture some of the electrons from this filled band. In silicon, the energy or band gap is about 1.1 V, so light can liberate an electron from the filled band, creating a free electron in the upper or conduction band, and a free hole in the lower or valence band. Vibrations in the crystal--heat--can also do this, so there are some free electrons in silicon even in the dark. As a practical matter, though, this intrinsic conduction is not seen. Impurities always dominate, making the material either n-type or p-type.
The diagram at the right shows the conditions in a semiconductor crystal at 0K. All the states in the valence band are full of electrons, absolutely all, so the electrons are packed so tightly they cannot move. All the states in the conduction band, at higher electron energy, are empty. The Fermi level is the energy at which a state is, on the average, occupied half the time. Above the Fermi level, the states are unoccupied, and below it, occupied, at T = 0K. The band gap energy Eg is the energy difference between the valence and conduction bands. Also shown is a donor site, perhaps As in Si, which has an extra electron easily kicked free, since it is not needed for the crystal binding. There is also an acceptor site, Al for example, that could use an extra electron to improve the crystal binding. At T = 0K, the electron is still in the donor, and the acceptor is still empty. The semiconductor is not a conductor at all, since there are no mobile charge carriers. The portion of the crystal with the donors will turn out to be n-type material, with movable electrons, and the part with the acceptors will turn out to be p-type material, with movable holes, when we heat the crystal up.
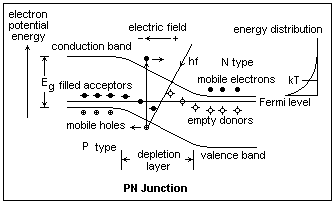With the addition of a little heat, the donors have ionized, and the donated electrons are now roaming around the conduction band. The acceptors have also ionized, grabbing electrons from the valence band, leaving holes that can roam about. The donors, fixed in the crystal, are now positively charged, while the acceptors are negatively charged. Nevertheless, the electrons and holes are exactly enough to balance the charges and things are still neutral. However, electrons may well bounce around to the left, and holes to the right, uncovering the fixed charges of the donors and acceptors. Since positive charges are uncovered on the right, and negative on the left, an electric field arises that forces the electrons and holes back where they belong. In the diagram, the Fermi level remains flat, but the bands are bent so that the Fermi level passes between the conduction band and the donor levels in the n-type material, and passes between the valence band and the acceptor levels in the p-type material. The bending of the bands corresponds exactly to the effect of the electric field on the electrons and holes. Some charge has left the region where the bands are sloping to expose the fixed positive and negative charges, and this has required a current to flow briefly, which charges this "capacitor." The region devoid of electrons and holes is called the depletion layer. In in, an electric field exists that sweeps out any mobile charges that may venture in.
In the energy diagram, electrons roll downhill but holes roll uphill where the bands slope. If light gives an electron in the valence band an energy hf greater than Eg, a free electron and a free hole are produced in this area, and are instantly swept out, creating a photocurrent.
It is very satisfying to prove to oneself that the PN junction forms a rectifier. In the conditions shown in the band diagram, there is no current through the junction. Actually, there are small currents both ways, but they cancel on the average. The electrons in the conduction band have kinetic energies as shown by the small graph on the right, that shows the thermal energy kT. This is the familiar exponential Boltzmann distribution. There is a similar situation with the holes, but it is not shown to avoid excess complication. If we make the barrier higher, by making the n-type material positive (since we are dealing with electron energies, it looks backwards, but electrons have a negative charge!). If we make the barrier lower, the upper part of the energy distribution of the electrons (and holes) peeks above the barrier, and charges can diffuse through. A current results, which increases exponentially because the Boltzmann distribution increases exponentially.
In a diode, the p region is called the anode, and the n region the cathode. Conduction occurs when the anode is made positive with respect to the cathode, and the current increases exponentially with the applied forward bias, as we well know. If we apply a large reverse bias, the strong electric field accelerates random charges that appear thermally, and if it is strong enough, gives the charges enough energy to knock out further charges. Breakdown then results, and the PN junction ceases to control the current. The heat developed usually destroys the device quickly when breakdown occurs.
With a reverse bias, there is only a small current produced by electron-hole pairs created in the depletion layer by thermal action. The strong electric field sweeps them out. When light falls on the depletion layer, pairs are copiously produced, and a considerable photocurrent results. For this to happen, light must both penetrate to the depletion layer, and also be absorbed there. These are antagonistic effects, so the actual photocurrent is a compromise. In silicon, the band gap of 1.1 eV corresponds to a wavelength of 1130 nm, and here absorption is greatest. As the wavelength decreases, the absorption also decreases, so more light can penetrate through bulk silicon to the depletion layer. However, less is proportionately absorbed, so the amount of light absorbed in the depletion layer decreases, passing on to be uselessly absorbed in the semiconductor beyond. In most practical silicon structures, the maximum sensitivity seems to occur around 850 nm. Other semiconductors also can be used, but silicon is so well known that it is employed for most photojunctions.
An electron and a hole can also recombine, the electron falling into the hole, as it were, releasing its energy, which will be greater than the energy gap. This energy can be released as light, but usually it is not, either because this is not favored by the band conditions (as in silicon) or because there are more efficient competing processes. In some semiconductors, such as gallium arsenide, GaAs, radiative recombination of electron-hole pairs is favored. When current passes through a depletion layer under forward bias, both electrons and holes can be made to take part, and when they encounter one another, light is produced. Since the energies of the electrons and holes are not far from the band limits at normal temperatures, the wavelength of the light corresponds to the energy gap. The wavelength of maximum emission is somewhat shorter than that corresponding to the gap, for some of the same reasons that displace the wavelength of maximum absorption. The light has to get out of the junction.
The band gap of GaAs is 1.4 V, so the emission limit is 885 nm. The peak of the emitted radiation is actually around 940 nm, with a width of about 50 nm (that is, most of the radiation is between 915 and 965 nm). This is a pretty good match with the peak sensitivity of silicon, and many emitters are made from GaAs. The band gap can be varied by adding Al to the mix, and emitters that match silicon closely at about 850 nm are available, but usually are not worth the bother. There is a wide variety of III-V semiconductors like GaAs that allows the tailoring of the band gap to any tastes. When the band gap is larger than about 1.8 V, the peak is in the visible. GaP, for example, with a band gap of 2.25 V, makes a green light-emitting diode (LED). LED's are now available in all colors from red to blue, with even some white LED's that mix colors. The working forward voltage of the diode increases as the wavelength decreases, an effect that is easily observed. LED's are made for greatest efficiency, watts of radiation per milliampere, not for high reverse breakdown voltage, so they should always be guarded against the application of a reverse voltage.
Optoelectronic Devices
The light path in an optoelectronic link can be in air or space between an emitter and detector in discrete packages, a link through a transparent medium such as an optical fibre, or between closely-spaced devices in a single package, in which the beam can be interrupted or reflected, or else in which the beam does not appear externally. The most-used optoelectronic devices are the LED and the optocoupler. In most applications, the light is simply another information path, and is not otherwise significant. Optoelectronics is also used to measure, monitor or study radiation, usually as discrete sources, and there are other forms of radiation detector that do not involve semiconductors. Among these detectors are the phototube and photomultiplier, the thermocouple and others, important in special applications. Here, we are mainly concerned with optoelectronics as circuit elements.
Emitters based on a pn-junction, like LED's, are all pretty much alike electrically. They require a series resistor to limit the current through them, and protection against reverse voltages. Distinguish between an LED (or IRED) and a photodiode, which is a pn-junction intended as a radiation detector, not a source.
As an example, I used a TIL31 GaAs IRED, rated at a maximum forward current of 200 mA and a reverse breakdown voltage of 2 V. (TIL is the prefix for Texas Instruments optoelectronics.) The connections to the TIL31, which comes is the premium TO-18 package, is shown at the right, together with those of the TIL99 phototransistor, mentioned below. You are looking at the bottom of the package, with the leads facing you. According to the specifications, this diode should give 6 mW of radiation at 100 mA, with a peak wavelength of 940 nm. I used a 100Ω series resistor, and found a voltage of 1.26 across the diode, which meant a current of 37 mA, which should produce a bit more than 2 mW of infrared. How can you tell it is working? Well, you can calibrate a detector for this purpose, but it would be nice to have some more direct method of sensing the infrared. I used a test card from Quantex, model Q-12-R that senses infrared. The card must be sensitized by exposing it to sunlight or fluorescent light for a while first. Then, infrared falling on it makes the coating glow, as if a light was falling on it, which is easy to see in dim light.
You can make tests with a variety of LED's of different colors, sizes and brightnesses, finding the forward voltages and how the forward voltage depends on the current. Of course, the eye is the detector here.
The most useful discrete receiver is a phototransistor. The base and collector of this device really make a photodiode, with a thin p layer on a thick n-type collector layer in the npn device, as shown in the figure. If you diffuse in an emitter region on the base, you can take advantage of transistor action to amplify the photocurrent greatly. The photocurrent is, in effect, base current supplied to the transistor, and is multiplied by beta. The only drawback is a rather long rise and fall time because of the large capacitance of the base, which is slow to charge and discharge. If you need speed, the much less sensitive photodiode is the only answer. In the figure, photon "a" is absorbed in the depletion layer, and contributes to the photocurrent. Photon "b" is absorbed in the neutral region of the collector, while photon "c" is absorbed in the neutral region of the base. Neither contribute to the photocurrent. The "real" base of the transistor is under the emitter, and most of the current flows here. The sensitive area penetrates deeper into the collector because the collector is more lightly doped than the base.
Since light must reach the base region, you can look into the package and see the device. In the TIL99, it is a golden-colored square, about a millimetre on a side, with two gold wires attached to it. The actual base probably looks transparent, and what you see is the heavily-doped region deep in the collector, which appears metallic. One of the wires is a connection with the base, while the other is the emitter, which occupies only a small area near one corner. The collector is connected to the metal encapsulation, as well as to a lead wire. The base connection need not be used, but is there so the device can be used as a photodiode if desired, and for convenience in some circuits. 
For testing as a phototransistor, I connected the collector with +5 through a 2.2k resistor, and grounded the emitter. With the ordinary desktop ambient light, this gave a current of 1.7 mA. As a photodiode, the collector is connected directly with +5, and the base to ground through a 1M resistor. With the same light, I measured a photocurrent of 2.2 μA (2.2 V across the resistor). This gives an effective beta of 773, rather larger than the specified minimum of 200. When the aperture is covered, small dark currents were measured. The load resistors used in the experiments were chosen to make measurements convenient. In practice, different values might be preferable, say a 100 Ω load with additional amplification, to gain speed and reduce dark current.
A curiosity, but a useful one, is the CdS photoconductive cell. CdS has a band gap of 2.42 V, so it can be expected to be most sensitive somewhere around 512 nm, close to the peak of visual sensitivity, and give a response similar to that of the eye. Its sensitivity extends out to 900 nm, and is most sensitive at 580 nm, so it is a better match to the eye than most photosensitive devices. It is not possible to make diodes and transistors from CdS, but a material intrinsic enough that light causes a significant increase in carrier density, and therefore a decrease in resistance, can be made. The cell I used has a resistance of about 3.3k in the dark, but only 232Ω under normal desktop illumination. It's easy to make a circuit that switches on an LED when the lights go out. The chief disadvantage of CdS is its slow response time; its time constant is about 50 ms. The chief advantage is the high sensitivity.
CdS Cell Characteristics
| E, ft-cd |
R, kΩ |
| 1 |
183 |
| 9 |
19 |
| 27 |
7.1 |
| 50 |
4.2 |
| 75 |
3.0 |
| 100 |
2.4 |
| 120 |
1.8 |
Another photoconductive cell circuit is shown at the right, and the measured characteristics of the cell at the left. This is a smaller cell than the one used above, and the element is in two parts with a center connection brought out. This enables the cell to detect motion of a spot of light from one half to the other half. In this case, the whole element is used and the center lead is left unconnected. It is clear that the cell can measure absolute values of illumination sensitively only below about 10 ft-cd. Above this point, the resistance varies much less rapidly with illumination. However, this makes the cell very good at distinguishing light from dark, as it is perceived. When the cell is put in a closed drawer, its resistance goes to 2.5 MΩ. The circuit shown gives an output of 4.9 V for the bright 120 ft-cd illumination of my desk, and 0.03 V when the light is turned off. Even the beam of a flashlight is easily detected. The hysteresis of the HC14 inverter is not really required here, but always gives better results when acquiring such external signals.
Discrete emitters and detectors are also furnished in special mountings that allow a 1 mm optical fibre to be connected as the light path. This is not the optical fibre that is used in communications, but a convenient light path for limited distances. One great advantage is the absence of conductive paths and the associated ground problems that often arise, as well as almost perfect isolation from interference. This 1 mm fibre is an excellent solution to many problems. Motorola, in particular, has supported it.
An interrupter module has an IRED in one part, and a phototransistor in the other, arranged so that the light path joining them can be obstructed by an object placed in the space between. The H22A1 is a typical interrupter module. The LED is on the side marked "E +" on the top. The "+"is over the lead connected to the anode of the diode, while the other lead is the cathode. This diode is rated at a maximum forward current of 60 mA, and is connected like any other LED, with a suitable current-limiting resistor. The phototransistor is on the side marked "+ D" on the top, with the lead to the collector under the "+" and the lead to the emitter under the D. It is rated at a maximum collector current of 100 mA, VCEO = 30 V, and VECO = 7 V. Using a 330Ω resistor and +5 V for the diode, and a 1k collector resistor to the same +5, I found a collector potential of 1.56 V. When the light path is obstructed, this rises to nearly 5 V.
We now come to the most-used optoelectronic device, the optocoupler. This device transfers signals without any electrical connection whatsoever, eliminating all problems arising from different ground references and different DC levels. The emitter and detector are contained within a sealed package, commonly a 6- or 4-pin DIP. A large variety of detectors is available, in addition to the phototransistor. These include a photodiode (for speed), a photoDarlington (for sensitivity), a photo-Schmitt-trigger for logic levels, a photo-FET (the resistance is controlled), a photo-SCR (for triggering), or a photo-triac (for AC triggering).
The 4N28 is a typical optocoupler, furnished in a 6-pin DIP. The diode has a maximum forward current of 80 mA and reverse voltage of 3 V. The phototransistor has maximum VCBO = 70V, VCEO = 30 V, VEBO = 7 V, and collector current 100 mA, all quite typical. All three connections are brought out to pins. The circuit can be wired up and tested quite easily, and the current transfer ratio determined. I got the ratio of the collector current to the diode current to be about 0.2. There are optocouplers with much better ratings; the 4N28 is an inexpensive type that will do good work in most cases. There is rarely any justification for using an expensive, premium device where a cheap one will do--they are all made the same way, and are equally reliable.
References
- General Electric Company, Optoelectronics, 2nd ed. (Auburn, NY: 1982). General Electric is now out of the semiconductor business, though once an innovative leader, and books like this one are no longer available. This is an excellent introduction, with specifications of many devices, from the golden age of electronics.
- Motorola, Optoelectronics Device Data (Phoenix, AZ: Motorola, 1993), or most recent edition. Like most data books, this one contains explanations of optoelectronic devices and application notes. Also see data books from other manufacturers, such as Texas Instruments and Hewlett-Packard.
Return to Electronics Index
Composed by J. B. Calvert
Created 6 July 2001
Last revised 29 July 2002